Is tan^2x1=secx a pythagorean identityThe Pythagorean identity tells us that no matter what the value of θ is, sin²θcos²θ is equal to 1 We can prove this identity using the Pythagorean theorem in the unit circle with x²y²=1 Created by Sal Khan Google Classroom Facebook TwitterFree multiple angle identities list multiple angleLearn how to solve trigonometric identities problems step by step online Prove the trigonometric identity 1tan(x)^2=sec(x)^2 Applying the trigonometric identity \tan(x)^21=\sec(x)^2 Since both sides of the equality are equal, we have proven the identity Get an answer for 'verify (1 tan^2x)/(tan^2x) = csc^2x' and find homework help for other Math questions at eNotes Verify the identity `1/(tan^2x) 1/(cot^2x) = csc^2x sec^2x` 2

Trig Identity Tan X 1 Tan 2 X Sec X Cos X Csc X Sin X Proved From Both Sides Youtube
Prove the identity 1+tan^2x/2tanx=csc2x
Prove the identity 1+tan^2x/2tanx=csc2x-2 x I started this by making sec 1/cos and using the double angle identity for that and it didn't work at all in any way ever Not sure why I can't do that, but something was wrong Anyways I looked at the solutions manual and they magic out 1 tan x tan 2 x = 1 tanI just want to ask The equation says tan2x=2tanx/1tan^2x How did it become 2tanx and 1tan^2x?



2
Simplify the expression using trig identities 1 (sin4x cos4x)/(sin2x cos2x) 2 (sinx(cotx)cosx)/(2cotx) Math Prove the identity sec^4x tan^4x = 12tan^2x Trig Use the fundamental identities to simplify the expression cot beta sec beta I used 1tan^2u=secu since cot is the inverse of tanB) (tanx 1) (tanx1)/1 tan^2 (x) =Math\sin^2x\cos^2x=1/math math\implies\dfrac{\sin^2x}{\cos^2x}\dfrac{\cos^2x}{\cos^2x}=\dfrac{1}{\cos^2x}/math math\implies\left(\dfrac{\sin x}{\cos x
A follow up proof to accompany sin^2 cos^2 =1 Another identity that is used quite a bit, especially in calculus involving trigonometric functionsTanx = t Sec^2 x dx= dt So now it is, 1/ (1t)^2 dt This integral is given by 1/1t and t= tanx So, it is cosx/cosx sinx tanx = t Sec^2 x dx= dt So now it is, 1/ (1t)^2 dt This integral is given by 1/1t and t= tanx So, it is cosx/cosx sinx Integral of the function \frac {\cos ^2 x} {1\tan x}Sec2 (x)(1 − sin2 (x)) = 1 sec 2 ( x) ( 1 sin 2 ( x)) = 1 Start on the left side sec2(x)(1−sin2(x)) sec 2 ( x) ( 1 sin 2 ( x)) Apply pythagorean identity sec2(x)cos2(x) sec 2 ( x) cos 2 ( x) Convert to sines and cosines Tap for more steps Apply the reciprocal identity to sec ( x) sec ( x)
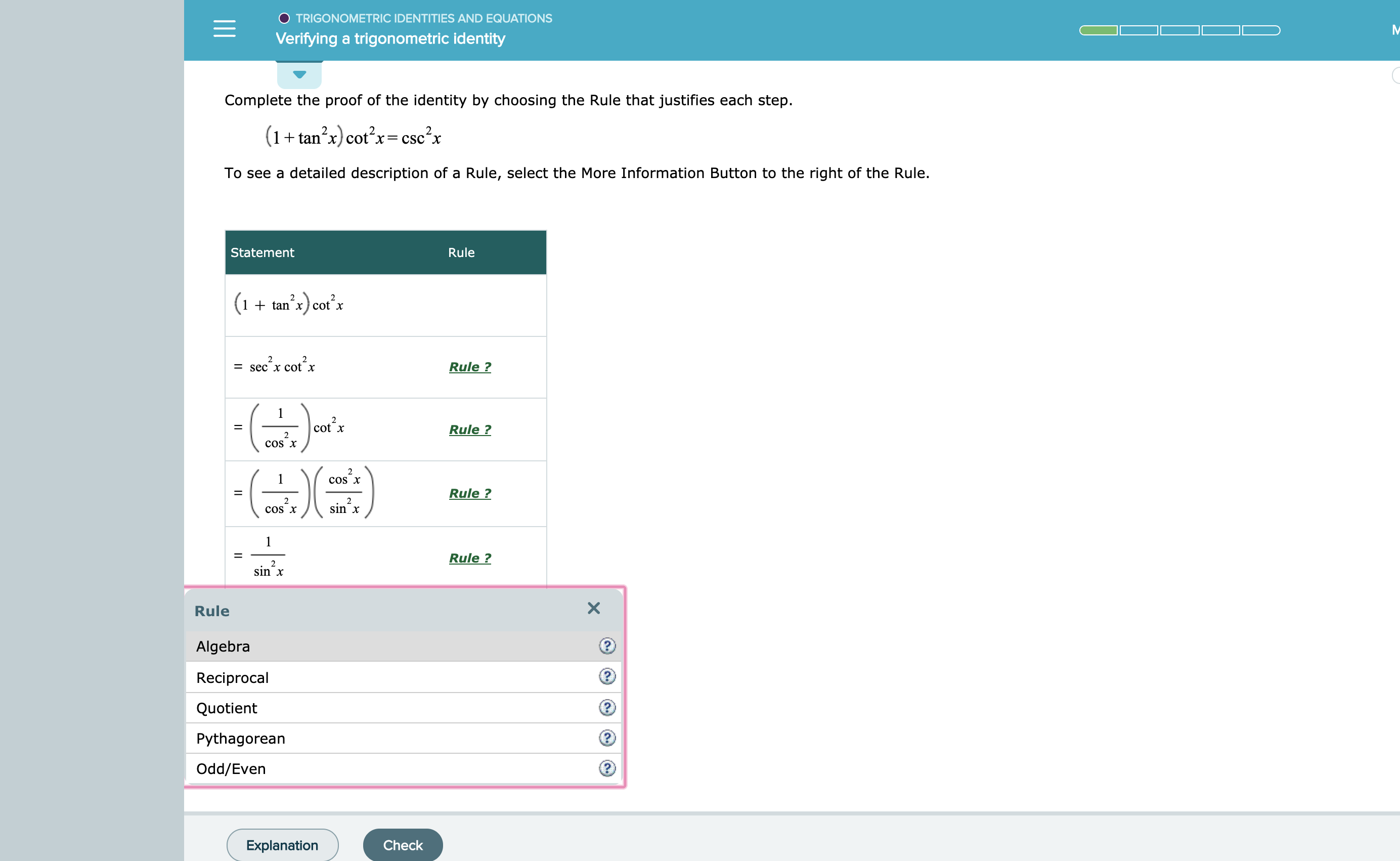
Answers Click here to see ALL problems on Trigonometrybasics Question I need to prove the identity (1tan^2x)cot^2x=csc^2x Found 2 solutions by Alan3354, Regrnoth Answer by Alan3354 () ( Show Source ) You can put this solution on YOUR website!Identities Proving Identities Trig Equations Trig Inequalities Evaluate Functions Simplify Statistics Arithmetic Mean Geometric Mean Quadratic Mean Median Mode Order Minimum Maximum Probability MidRange Range Standard Deviation Variance Lower Quartile Upper Quartile Interquartile Range Midhinge Standard Normal Distribution 1tan^2(x) = 1 (sin2x)/(cos2x) = cos2x sin2x/cos2x = cos 2x/cos2x is a posibly 'simplified' version in that it has been boiled down to only cosines



Www Birmingham K12 Mi Us Cms Lib Mi Centricity Domain 976 Chapter 5 Pdf




Tan 2x Tan 2x
I know that and The next step would then be to say that but now what?1 sin 2x = 1 sin 2x (Pythagorean identity) Therefore, 1 sin 2x = 1 sin 2x, is verifiable HalfAngle Identities The alternative form of doubleangle identities are the halfangle identities Sine • To achieve the identity for sine, we start by using a doubleangle identityThese identities are useful whenever expressions involving trigonometric functions need to be simplified An important application is the integration of nontrigonometric functions a common technique involves first using the substitution rule with a trigonometric function, and then simplifying the resulting integral with a trigonometric identity




Solved Verify Each Identity Solve As A Two Column Proof Chegg Com




Ex 3 3 23 Prove Tan 4x 4 Tan X 1 Tan2 X 1 6tan2x
The trigonometric identity `(tan^2x)/(1tan^2x) = sin^2x` has to be proved Start with the left hand side `(tan^2x)/(1tan^2x)` Substitute `tanx = sin x/cos x`Learn how to solve trigonometric identities problems step by step online Prove the trigonometric identity 1tan(x)^2=sec(x)^2 Applying the trigonometric identity \tan(x)^21=\sec(x)^2 Since both sides of the equality are equal, we have proven the identityVerify this identity (tan^2 (x)1)/ (1tan^2 (x)) = 12cos^2 (x) I've started a couple different options but none are working out for me Here is what I have so far A) multiply top by (1 tan^2 (x)) to get tan^4 (x) 1 or (tan^2 (x)1) (tan^2x1) then i'm stuck!



1




How Do You Prove The Identity Tan 2x Secx 1 1 Cosx Cosx Socratic
Prove the identity 1tan^2x/2tanx=csc2x1 1 Because the two sides have been shown to be equivalent, the equation is an identity cos2(x)(1tan2(x)) = 1 cos 2 (x) (1 tan 2 (x)) = 1 is an identity1 cos ( x) − cos ( x) 1 sin ( x) = tan ( x) Go!Verifying the identities illustrates how expressions can be rewritten to simplify a problem Simplifying one side of the equation to equal the other side is a method for verifying an identity See Example \(\PageIndex{1}\) and Example \(\PageIndex{2}\) The approach to verifying an identity depends on the nature of the identityTrigonometric identities Intro to Pythagorean trigonometric identities Converting between trigonometric ratios example write all ratios in terms of sine Practice Evaluating expressions using basic trigonometric identities Trigonometric identity example proof involving sec, sin, and cos



Answered Trigonometric Identities And Equations Bartleby



Www2 Math Binghamton Edu Lib Exe Fetch Php People Mckenzie Trig Identities Worksheet With Answers 2 Pdf
Introduction to Tan double angle formula let's look at trigonometric formulae also called as the double angle formulae having double angles Derive Double Angle Formulae for Tan 2 Theta \(Tan 2x =\frac{2tan x}{1tan^{2}x} \) let's recall the addition formulaCorrect answers 3 question Verify the identity (1 tan^2x)(1 sin^2x) = 1 i know the solution, but not the steps to get me the answers i need i will need to know how to do this on my own for a test soon, so any explanations will be appreciated solution, kinda (1 tan^2x)(1 sin^2x) = sex^2x * cos^2x = 1/cos^2x = 1 here are some identities that i'm pretty sure need to be used, but i'mProve the identity 2tanx/1tan^2x=sin^2x 11,8 results, page 18 trig If sin theta is equal to 5/13 and theta is an angle in quadrant II find the value of cos theta, sec theta, tan theta, csc theta, cot theta Math Find the values of the six trigonometric functions of
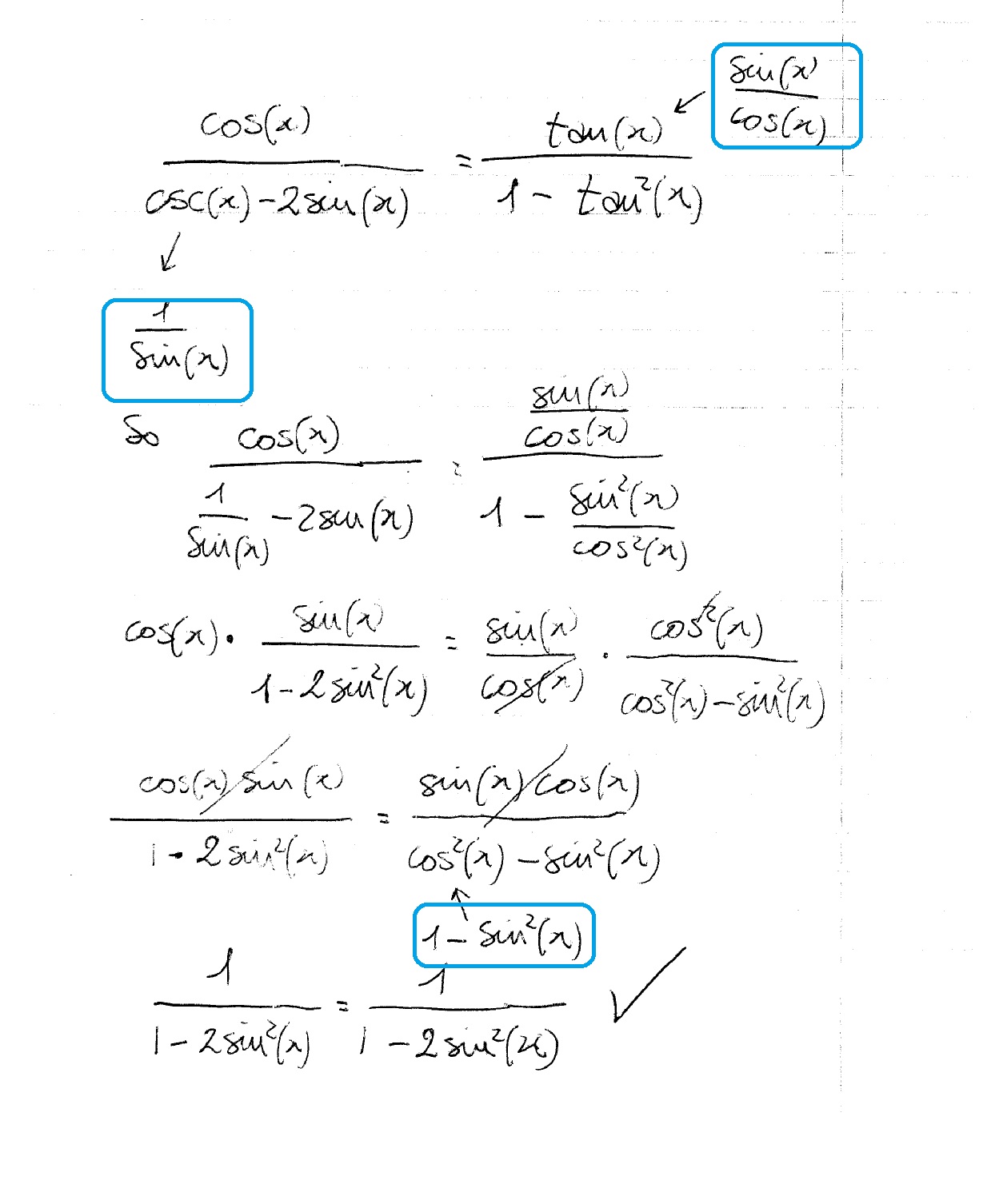



How Do You Prove Cosx Cscx 2sinx Tanx 1 Tan 2x Socratic



Wl Apsva Us Wp Content Uploads Sites 38 15 05 Lesson 6 4 Notes 1 Pdf



0 件のコメント:
コメントを投稿